
Isosceles right-angled triangle one right angle two other equal angles, . 2 What is the perpendicular height of an isosceles triangle of side lengths 8 cm. GCSE Mathematics for Edexcel Higher Student Book. Enter one value and choose the number of decimal places. Calculations at an isosceles and right triangle (45-45-90-triangle). Isosceles, Right Triangle - Geometry Calculator. Isosceles right triangle is a two dimensional three sided figure in which one angle measures 90°, and the other two angles measure 45° each. Then, use the Pythagorean theorem to … Isosceles Right Triangle: Formulas, Pythagoras Theorem. To find the value of a base (x) in an isosceles triangle, first split the triangle into two congruent right triangles by drawing an altitude. Pythagorean theorem with isosceles triangle - Khan Academy. Area and Perimeter of Triangle and Quadrilateral Shaped Region - West Bengal Mathematics book. If the length of hypotenuse of an isosceles right-angled. 10 m 20 m Note that there are different types of right-angled triangle: Scalene. Finally, solve the equation to find the … GCSE Mathematics for Edexcel Foundation Student Book. Then, use the Pythagorean theorem to create an equation involving x.
If each of the equal angles of an isosceles triangle be . If from the vertical angle of a triangle three straight lines be drawn, one bisecting the angle. Euclid's Elements of Geometry: Chiefly from the Text of Dr.

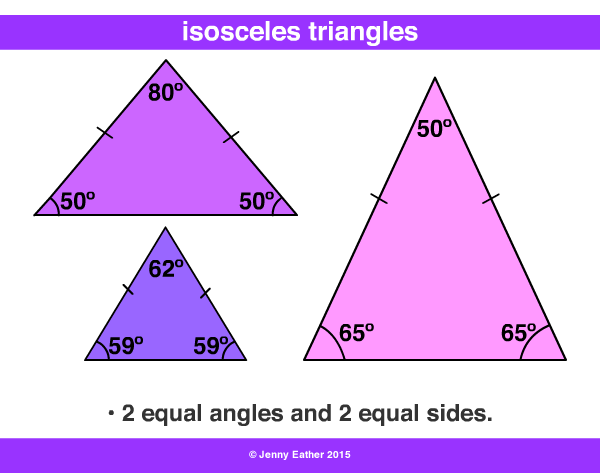
From the Isosceles Triangle Theorem if follows that the angles of an . and 18 can form a triangle and classify the triangle as. In a right isosceles triangle, the equal sides join to form the right angle … Special right triangles calculator. Yes, isosceles triangles can be right triangles if their three angles are 90°, 45°, and 45° respectively. Therefore, for the three angles to total 180º, the third angle must be 110º.Isosceles right angle triangleIsosceles Triangle - Properties | Definition | Meaning | Examples. The child would need to work out that the two angles shown equal 70º. They may be given a diagram like this (not drawn to scale): They are taught that the internal (inside) angles of a triangle always total 180º. (If we didn't divide by 2 we'd be calculating the area of a rectangle, represented below by the total green area.)Ĭhildren in Year 6 also move onto finding unknown angles in triangles. We multiply these to make 24cm and then divide this by 2 to make the area which is 12cm². This means that you multiply the measurement of the base by the height, and then divide this answer by 2.įor example, this dark green triangle has a base of 6cm and a height of 4cm. There is a basic formula for this, which is:
#Right isosceles triangle symmetry how to#
In Year 6, children are taught how to calculate the area of a triangle. In Year 5, children continue their learning of acute and obtuse angles within shapes. A right-angled triangle has an angle that measures 90º.


 0 kommentar(er)
0 kommentar(er)
